Next: Second Problem Set: more Up: MATH 2400 INTRODUCTION TO Previous: Final Project
Since latex2htm program chokes on some equations, I have put the course webpage to LMS in pdf format
Find the general solutions of the following first-order linear differential equations:
1.2.
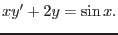
3.4.
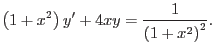
Find the solutions to the following problems:
5.6.

7.8.
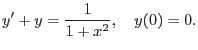
9. Find the general solution of the equation
Find the solutions of the given differential equations:
10.11.
12.
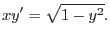
Find the explicit solutions to the initial value
problems:
13.14.
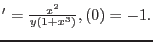
15. Einsteinium-253 decays at a rate proportional to the
amount present. Determine the half-life ![]() , if this
material loses one third of its mass in 11.7 days.
, if this
material loses one third of its mass in 11.7 days.
16. Suppose that 100 mg of thorium-234 are initially
present in a closed container, and that thorium-234 is
added to the container at a constant rate of 1
mg/day.
(a) Find the amount ![]() of thorium-234 in
the container at any time, given that its decay rate is
0.02828 days
of thorium-234 in
the container at any time, given that its decay rate is
0.02828 days![]() .
.
(b) Find the limiting amount ![]() of thorium-234 in the
container as
of thorium-234 in the
container as ![]() .
.
(c) How long a time period must elapse before the amount
of thorium-234 in the container drops to within 0.5 mg of
the limiting value ![]() ?
?
(d) If thorium-234 is added to the container at a rate of
![]() mg/day, find the value of
mg/day, find the value of ![]() that is required
tomaintain a constant level of 100 mg of thorium-234.
that is required
tomaintain a constant level of 100 mg of thorium-234.
17. A tank initially contains 120 liters of pure water.
A mixture containing ![]() g/liter of salt enters the
tank at a rate 2 liters/min, and the well-stirred mixture
leaves the tank at the same rate. Find an expression in
terms of
g/liter of salt enters the
tank at a rate 2 liters/min, and the well-stirred mixture
leaves the tank at the same rate. Find an expression in
terms of ![]() for the amount of salt in the tank at
any time
for the amount of salt in the tank at
any time ![]() . Also, find the limiting amount of salt in
the tank as
. Also, find the limiting amount of salt in
the tank as ![]() .
.
18. Suppose that a room containing 120 ft![]() of air
is originally free of carbon monoxide. Beginning at time
of air
is originally free of carbon monoxide. Beginning at time
![]() cigarette smoke, containing 4% of carbon monoxide,
is introduced to the room at a rate of 0.1 ft
cigarette smoke, containing 4% of carbon monoxide,
is introduced to the room at a rate of 0.1 ft![]() /min,
and the well-circulated mixture is allowed to leave the
room at the same rate.
/min,
and the well-circulated mixture is allowed to leave the
room at the same rate.
(a) Find an expression for the concentration ![]() of
carbon monoxide in the room at any time
of
carbon monoxide in the room at any time ![]() .
.
(b) Extended exposure to a carbon monoxide concentration
as low as 0.00012 is harmful to the human body. Find the
time ![]() at which this concentration is reached.
at which this concentration is reached.
In each of the following two problems sketch ![]() versus
versus
![]() , determine the equilibrium points and classify each
one as stable or unstable:
, determine the equilibrium points and classify each
one as stable or unstable:
19.20.
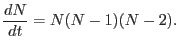
21. Semistable Equilibrium Solutions: Sometimes a
constant equilibrium solution has the property that
solutions lying on one side of the equilibrium solution
tend to approach it, whereas solutions lying on the other
side recede from it. In this case the equilibrium
solution is said to be semistable.
(a) Consider the equation
In each of the following two problems sketch ![]() versus
versus
![]() and determine the equilibrium points. Also classify
each equilibrium point as stable, unstable, or semistable:
and determine the equilibrium points. Also classify
each equilibrium point as stable, unstable, or semistable:
22.23.
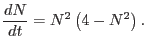
24. Consider the following model of a fishery. Let us
assume that the fish are caught at a constant rate ![]() independent of the size of the fish population
independent of the size of the fish population ![]() .
Then
.
Then ![]() satisfies the equation
satisfies the equation
In each of the following problems find the general solution of the given differential equation:
25.26.
27.
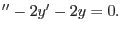
28. Find the solution of the initial value problem
In each of the following problems find the general solution of the given differential equation:
29.30.
31.
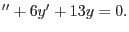
32. Find the solution of the initial value problem
In each of the following two problems find the general solution of the given differential equation:
33.34.
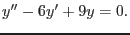
Find the solution of the following initial value
problems:
35.
![]()
36.
![]()
37.
![]()
In the following problems, determine the suitable form for the particular solution. You do not need to
evaluate the constants.
38.
![]()
39.
![]()
40.
![]()
In the following three problems, find the general solution to the given differential equation:
41.42.
43.
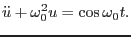
In the following problems, determine the suitable form for the particular solution. You do not need to
evaluate the constants.
44.
![]()
45.
![]()
In each of the following problems, given one solution,
use reduction of order to find the general solution of the
given differential equation:
46.
![]()
47.
![]()
48.
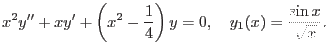
Find the general solutions of the following Euler's equations
49.50.
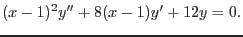
51.52.
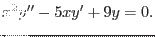
53. Find the solution of the initial-value problem
In each of the following problems use variation of parameters to find the general solution of the given differential equation:
54.55.
56.
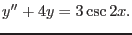
57. Find the general solution of the differential
equation
In each of the following two problems determine
![]() ,
, ![]() , and
, and ![]() so as to write the given
expression in the form
so as to write the given
expression in the form
![]()
58.59.
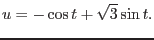
60. A mass weighing 16 lb stretches a spring 3 in. The
mass is attached to a viscous damper with a damping
constant of 2 lb-sec/ft. If the mass is set in motion
from its equilibrium position with a downward velocity of
3 in/sec, find its position ![]() at any time
at any time ![]() . Draw
. Draw ![]() versus
versus ![]() . Determine when the mass first returns to its
equilibrium position. Also, find the time
. Determine when the mass first returns to its
equilibrium position. Also, find the time ![]() such
that
such
that ![]() in for all
in for all ![]() . (Recall that
. (Recall that ![]() ft/sec
ft/sec![]() .)
.)
The positions of certain mass-spring systems satisfy the following initial value problems
61.(a) Find the solutions of these initial value problems.62.
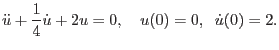
63. A mass of 5 kg stretches a spring 10 cm. The mass is
acted on by an external force of ![]() N
(newtons=kg
N
(newtons=kg![]() m/sec
m/sec![]() ) and moves in a medium that
imparts a viscous force of 2 N when the speed is of the
mass is 4 cm/sec.
) and moves in a medium that
imparts a viscous force of 2 N when the speed is of the
mass is 4 cm/sec.
(a) If the mass is set in motion from its
equilibrium position with an initial velocity of 3 cm/sec,
formulate the initial value problem describing the motion
of the mass, and find its solution.
(b) Identify the transient and steady-state parts of the
solution.
(c) Draw the graph of the solution, as well as the
steady-state solution.
(d) If the given external source is replaced by a force
![]() of frequency
of frequency ![]() , find the value of
, find the value of
![]() for which the amplitude of the forced response is
maximum.
for which the amplitude of the forced response is
maximum.
64. Consider a vibrating system described by the initial
value problem
In each of the following problems, find the eigenvalues and eigenfunctions of the given boundary-value problem. Assume that all eigenvalues are real.
65.66.

In each of the following problems, find the Fourier series corresponding to the given function:
67.
![$\displaystyle f(x)=\left\{\begin{array}{ll}1,\qquad
&-l\leq x <0, [6pt] 0, & \;\; 0\leq x
<l,\end{array}\right. \qquad f(x+2l)=f(x).$](img129.png)
68.
![]()
69.
![$\displaystyle f(x)=\left\{\begin{array}{ll}x+1,\qquad
&-1\leq x <0, [6pt] x, & \;\; 0\leq x
<1,\end{array}\right. \qquad f(x+2)=f(x).$](img131.png)
70.
![$\displaystyle f(x)=\left\{\begin{array}{ll}x+1,\qquad
&-1\leq x <0, [6pt] 1-x, & \;\; 0\leq x
<1,\end{array}\right. \qquad f(x+2)=f(x).$](img132.png)
71.
![$\displaystyle f(x)=\left\{\begin{array}{ll}x+l,\qquad
&-l\leq x \leq 0, [6pt] l, & \;\; 0< x
<l,\end{array}\right. \qquad f(x+2l)=f(x).$](img133.png)
72. If ![]() for
for ![]() and
and ![]() , find a
formula for
, find a
formula for ![]() in the interval
in the interval ![]() , and in the
interval
, and in the
interval ![]() .
.
Find the Fourier series for the following problems. Assume that the functions are periodically extended outside the original interval. Sketch the function to which each series converges outside the original interval.
73.
![$\displaystyle f(x)=\left\{\begin{array}{ll}1,\qquad
&\qquad 0\leq x<s <1, [6pt] 0, & \qquad s\leq x<2-s,
[6pt] 1, & 2-s \leq x <2.\end{array}\right. $](img140.png)
74.
![]() .
.
75.
![$\displaystyle f(x)=\left\{\begin{array}{ll}0,\qquad
&-1\leq x <0, [6pt] x^2, & \;\; 0\leq x
<1.\end{array}\right.$](img142.png)
In each of the following problems find the required Fourier series for the given function; sketch the graph of the function to which the function converges over two or three periods.
76.
![$\displaystyle f(x)=\left\{\begin{array}{ll}1-x,
\qquad &0< x \leq 1, [6pt] 0, &1 < x
\leq 2.\end{array}\right.\quad$](img143.png) Both even and odd
extensions, period 4.
Both even and odd
extensions, period 4.
77.
![$\displaystyle f(x)=\left\{\begin{array}{ll}x,
\qquad &0\leq x < 1, [6pt] 1, &1 \leq x
< 2.\end{array}\right.\quad$](img144.png) Sine series, period 4.
Sine series, period 4.
78.
![]() Cosine series, period
Cosine series, period ![]() .
.
79.
![]() Sine series, period
Sine series, period ![]() .
.
80.
![]() Cosine series, period
Cosine series, period ![]() .
.
81.
![]() Sine series, period
Sine series, period ![]() .
.
82. State exactly the boundary value problem determining
the temperature in a silver rod 2 meters long if the ends
are held at the temperatures 30![]() Celsius and
50
Celsius and
50![]() Celsius, respectively. The thermal diffusivity
of silver is
Celsius, respectively. The thermal diffusivity
of silver is
![]() cm
cm![]() sec. Assume that the
initial temperature in the bar is given by a quadratic
function of the distance along the bar consistent with the
preceding boundary conditions, and with the condition that
the temperature at the center of the rod is 60
sec. Assume that the
initial temperature in the bar is given by a quadratic
function of the distance along the bar consistent with the
preceding boundary conditions, and with the condition that
the temperature at the center of the rod is 60![]() Celsius.
Celsius.
83. Find the solution of the heat conduction problem
In each of the following problems determine whether the method of separation of variables can be used to replace the given partial differential equation by a pair of ordinary differential equations. If so, find the equations.
84.85.
86.
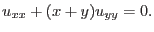
87. Consider the conduction of heat in a copper rod 100
cm in length whose ends are maintained at 0![]() Celsius for all
Celsius for all ![]() . Find an expression for the
temperature
. Find an expression for the
temperature ![]() if the initial temperature
distribution in the rod is given by
if the initial temperature
distribution in the rod is given by
![\begin{displaymath}u(x,0)=\left\{\begin{array}{ll}0, \qquad & 0\leq x
<25 [3pt...
...25\leq x \leq 75 [3pt]
0, & 75 < x \leq 100\end{array}\right.\end{displaymath}](img161.png)
88. Let a metallic rod 20 cm long be heated to a uniform
temperature of 100![]() Celsius. Suppose that at
Celsius. Suppose that at ![]() the ends of the bar are plunged into an ice bath at
0
the ends of the bar are plunged into an ice bath at
0![]() Celsius, and thereafter maintained at this
temperature, but that no heat is allowed to escape through
the lateral surface. Find an expression for the
temperature at any point in the bar at any later time.
Use two terms in the series expansion to determine
approximately the temperature at the center of the bar at
time
Celsius, and thereafter maintained at this
temperature, but that no heat is allowed to escape through
the lateral surface. Find an expression for the
temperature at any point in the bar at any later time.
Use two terms in the series expansion to determine
approximately the temperature at the center of the bar at
time ![]() sec if the bar is made of (a) silver, (b)
aluminum, or (c) cast iron.
Thermal diffusivities of silver, aluminum, and cast iron
are 1.71 cm
sec if the bar is made of (a) silver, (b)
aluminum, or (c) cast iron.
Thermal diffusivities of silver, aluminum, and cast iron
are 1.71 cm![]() /sec, 0.86 cm
/sec, 0.86 cm![]() /sec, and 0.12 cm
/sec, and 0.12 cm![]() /sec,
respectively. Also, use just one term in the
series expansion for
/sec,
respectively. Also, use just one term in the
series expansion for ![]() to find the time that will
elapse before the center of the bar cools to a temperature
of 25
to find the time that will
elapse before the center of the bar cools to a temperature
of 25![]() Celsius for each of the three metals.
Celsius for each of the three metals.
89. Let an aluminum rod of length ![]() be initially at the
uniform temperature of 25
be initially at the
uniform temperature of 25![]() Celsius. Suppose that
at time
Celsius. Suppose that
at time ![]() the end
the end ![]() is cooled to 0
is cooled to 0![]() Celsius, while the end
Celsius, while the end ![]() is heated to 60
is heated to 60![]() Celsius, and both are thereafter maintained at those
temperatures.
Celsius, and both are thereafter maintained at those
temperatures.
(a) Find the temperature distribution in the rod at any
time ![]() .
.
Assume in the remaining parts of this problem that ![]() cm.
cm.
(b) Use only the first term in the series for the
temperature ![]() to find the approximate temeprature
at
to find the approximate temeprature
at ![]() cm when
cm when ![]() sec; when
sec; when ![]() sec.
sec.
(c) Use the first two terms in the series for ![]() to
find an approximate value of
to
find an approximate value of ![]() . What is the
percentage difference between the one- and -two-term
approximations? Does the third term have any appreciable
effect for this value of
. What is the
percentage difference between the one- and -two-term
approximations? Does the third term have any appreciable
effect for this value of ![]() ?
?
(d) Use the first term in the series for ![]() to
estimate the time interval that must elapse before the
temperature at
to
estimate the time interval that must elapse before the
temperature at ![]() cm comes within 1 percent of its
steady state value.
cm comes within 1 percent of its
steady state value.
90. Consider a uniform rod of length ![]() with an initial
temperature given by
with an initial
temperature given by ![]() ,
, ![]() .
Assume that both ends of the bar are insulated. Find the
temperature
.
Assume that both ends of the bar are insulated. Find the
temperature ![]() , and the steady-state temperature as
, and the steady-state temperature as
![]() .
.
In each of the following problems find the
steady-state solution of the heat conduction equation
![]() that satisfies the given set of
boundary conditions.
that satisfies the given set of
boundary conditions.
91.92.
93.

94. Find the steady-state solution of the partial
differential equation
95. Consider a uniform bar of length ![]() having an
initial temperature distribution given by
having an
initial temperature distribution given by ![]() ,
,
![]() . Assume that the temperature at the end
. Assume that the temperature at the end
![]() is held at 0
is held at 0![]() Celsius, while the end
Celsius, while the end ![]() is
insulated so that no heat passes through it.
is
insulated so that no heat passes through it.
(a) Show that the fundamental solutions of the partial
differential equation and boundary conditions are
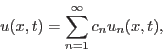
96. Find the displacement ![]() in an elastic string,
fixed at both ends, that is set in motion with no initial
velocity from the initial position
in an elastic string,
fixed at both ends, that is set in motion with no initial
velocity from the initial position ![]() , where
, where
![\begin{displaymath}f(x)=\left\{\begin{array}{ll}Ax, \quad\qquad & 0\leq x
\leq l...
... x < 3l/4 [3pt]
A(l-x), & 3l/4\leq x\leq l.\end{array}\right.\end{displaymath}](img181.png)
97. Find the displacement ![]() in an elastic string of
length
in an elastic string of
length ![]() , fixed at both ends, that is set in motion from
its straight equilibrium position with the initial
velocity
, fixed at both ends, that is set in motion from
its straight equilibrium position with the initial
velocity ![]() defined by
defined by
![\begin{displaymath}g(x)=\left\{\begin{array}{ll}Ax, \quad\qquad & 0\leq x
\leq l/2 [3pt]
A(l-x), & l/2\leq x\leq l.\end{array}\right.\end{displaymath}](img183.png)
98. If an elastic string is free at one end, the boundary
condition there is that ![]() . Find the displacement in
an elastic string of length
. Find the displacement in
an elastic string of length ![]() , fixed at
, fixed at ![]() and free
at
and free
at ![]() , set in motion with no inital velocity from the
initial position
, set in motion with no inital velocity from the
initial position ![]() , where
, where ![]() is a given
function.
is a given
function.
HINT: Show that the fundamental solutions for this
problem, satisfying all conditions except the
inhomogeneous initial condition, are
99. A vibrating string moving in an elastic medium
satisfies the equation
100. The motion of a circular elastic membrane, such as a
drum head, is governed by the two-dimensional wave
equation in polar coordinates
In each of the following problems find the general solution of the given system of equations. Also, sketch a number of representative trajectories.
101.102.
103.
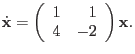
104.105.
106.
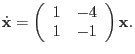
107.108.
109.
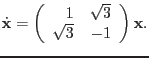
In each of the following problems find all the
equilibrium points, determine their type and stability,
and sketch local phase portraits near them. If possible,
also try to sketch the global phase portraits in the whole
![]() plane.
plane.
110.
![]()
111.
![]()
112.
![]()
113.
![]()
114.
![]()
115.
![]()
Dr Yuri V Lvov 2017-12-10